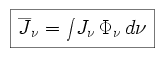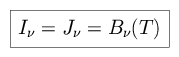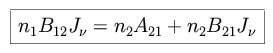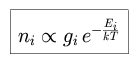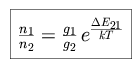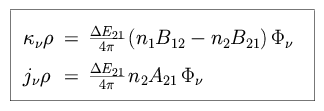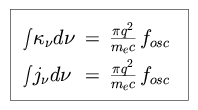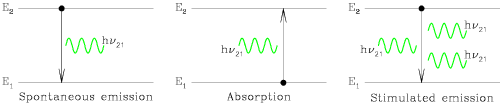
There are three Einstein coefficients, B₁₂, B₂₁, and A₂₁, which are used to calculate the probability per unit time for emission or absorption of a photon from an energy level within an atom.

Spontaneous emission: A₂₁ is the probability per unit time for spontaneous emission of a photon, moving an electron from the upper level to the lower level. It may be calculated from first principles, using quantum mechanics.

Absorption: B₁₂ × J is the probability per unit time for absorption of a photon, moving an electron from the lower level 1 to the upper level 2.

Stimulated emission: B₂₁ × J is the probability per unit time for stimulated emission, moving an electron from the upper level to the lower level.

[NMSU, N. Vogt]

Because the line profile has a finite width, the mean intensity J should be calculated for this purpose by integrating over the width of the line profile in frequency space:

For atoms in a state of thermodynamic equilibrium, there are three conditions which are met.

• The radiation field can be expressed as

• There is an equilibrium condition between the rate of absorption and the rate of emission. We define n_i as the number density of electrons in energy state i, and then require that

• The number density of electrons in energy state i is determined by the temperature of the gas. Defining g_i as the statistical weight (i.e. the degeneracy) of state i,

The ratio of the electron density between levels is then given by

We observe that at low temperatures, most electrons are found in the ground state, while at high temperatures the higher energy levels will be populated. The mass absorption and emission coefficients, in units of erg/gm/s/str/Hz, become

Furthermore, defining the oscillator strength of the transition,