Sidereal Time:
The rotation of the Earth causes a regular change in the apparent positions of stars, so the hour angle of a star can be used as a measure of time. We use the hour angle of the first point of Aries, HA, as a measure of the rotation of the Earth with respect to the stars. This is known as local sidereal time:

LST = HA

and is zero when the first point of Aries crosses the observer's meridian. As its name implies, local sidereal time depends upon the observer's longitude on the Earth's surface. The HA is equal to the sum of the right ascension of the star X (RAX) and the hour angle of the star X (HAX). Hence,

This is a very important relationship because X can be any celestial object - star, Sun, Moon, planet or spacecraft. Since the hour angle of a star is zero when it transits on the observer's meridian, the star's right ascension at that time is the local sidereal time (or, equivalently, the local sidereal time gives the right ascension of the star).

Solar Time:
Civil time keeping is based on the hour angle of the Sun (HAS) instead of the hour angle of the first point of Aries. This is known as apparent solar time (AST):

and is zero when the Sun crosses the observer's meridian. An apparent solar day is defined as the time interval between successive passages of the Sun across the observers' meridian. The time it takes the Sun to return to the same point in the sky each day is slightly longer than one sidereal day, which is the time it takes the stars to return to the same point in the sky each night.

[NMSU, N. Vogt]

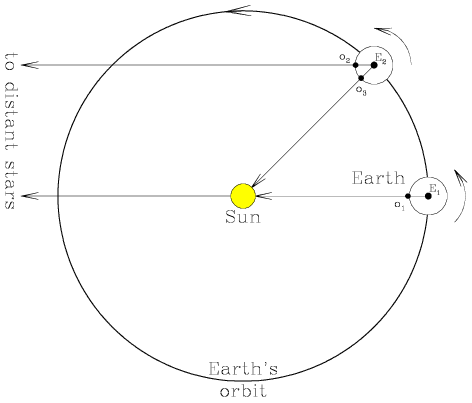
An observer at O₁ on the surface of the Earth observes the Sun and a star on the meridian. Taking the distance of the star to be effectively infinite compared to the Sun, the observer will next observe the star to transit at O₂ when the Earth has rotated once on its axis. However, in the time the Earth has taken to rotate once on its axis, it has also moved in its orbit around the Sun by 360/365.25=0.99°, from position E₁ to E₂. The Earth will thus have to rotate on its axis until the observer is at O₃ for the Sun to appear again on the meridian. The apparent solar day is therefore longer than the sidereal day by a time interval equal to the time it takes the Earth to rotate on its axis by the angle 360°/365.25, i.e. approximately four minutes. Given that an apparent solar day is 24 solar hours in length, this means that a sidereal day is only 23^h56^m long in solar units and represents the rotational period of the Earth. Another way of expressing this is that the Sun appears to move backwards through the stars by four minutes (or 1°) each day.

Each type of day (apparent solar and sidereal) can be divided into hours, minutes and seconds, but the solar version of each is 24^h/23^h56^m = 1.0028 times or 0.28% longer than the sidereal equivalent.

Universal Time:
The hour angle of the sun depends on the location of the observer and varies with longitude. It is therefore more convenient and less ambiguous to refer to a standard longitude, which was chosen by international agreement in 1884 to be the longitude of the old Royal Observatory at Greenwich. We can then define Greenwich mean time (GMT), or universal time, in terms of the hour angle of the sun at Greenwich (GHAMS):

UT = GMT = GHAMS +/- 12^h

where the plus or minus sign is used if the GHAMS is less than or greater than 12^h, respectively, in order to make the UT 0^h at midnight. For convenience, time within a particular country or geographical region is defined by time zones, 15° wide in longitude, within each of which the time is the same (the mean solar time at the center of the zone). The standard time or zone time is then defined by:

ST = ZT = UT + n

where n is given in hours and is a constant for a particular time zone, being negative for western longitudes and positive for eastern ones:

n = (longitude in degrees at center of zone) / 15

In most cases n is taken to be an integer, so that different time zones are an exact number of hours apart. An exception is India, where n=5.5. For geographical reasons, the time-zone borders do not always follow lines of longitude; for example, the International Date Line, which marks the center of the zones +/-12, snakes its way around groups of Pacific islands so that all islands in a group agree on the date.

Before the advent of accurate clocks, the rotation of the Earth was the fundamental time keeper for all purposes, both astronomical and civil. As soon as clocks became precise enough to detect fluctuations in the rotation rate of the Earth, the importance of astronomical observations for civil time keeping began to diminish and nowadays intervals of time are defined by atomic clocks, the corresponding time scale being known as coordinated universal time. This drifts slowly with respect to UT, because of fluctuations in the Earth's rotation rate, and leap seconds are added from time to time to keep UTC in phase with UT. Otherwise, (civil) noon would eventually be at (astronomical) midnight. Thus we still rely on astronomical observations to keep the zero-point of our timescales correct.

Ephemeris Time:
Visualize the planar surface in which Jupiter's orbit lies. We can measure the moment at which the Earth crosses this plane, twice per orbit, and define a unit of ephemeral time as the interval between successive crossings in the same direction (e.g. successive breaking of the plane from below, or successive breaking of the plane from above).

Atomic Time:
Consider the cesium isotope ¹³³Cs, which has a ground state transition between two hyperfine levels. We can measure the frequency of the radiation emitted during this transition, and the period of this radio wave serves as a unit of atomic time.

Nuclear Time:
We know that ³H will beta decay into ³He. If the tritium is cooled to 10 K, the helium will diffuse outward as it forms. When the mass of the tritium sample drops to half of its initial value, one unit of nuclear time has elapsed.

Pulsar Time:
There exist binary pulsars which emit signals with a periodicity, resulting from the neutron star rotation, which varies by less than one part in ten billion per year.

We can measure time by tracking inertial processes (the Earth's rotational rate), gravitational force (orbital patterns), electromagnetic processes, or nuclear beta decay (weak force) and alpha decay (strong force). Universal and ephemeral time should be related, if general relativity holds; should we assume that the time measured by all of these processes is equivalent?