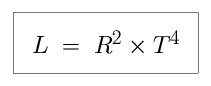What kind of stars are found here?

A star in this region of the Hertzsprung-Russell diagram has a temperature of roughly 20,000 kelvin (20,000 K), a luminosity 3,000 times greater than that of the Sun (3,000 × LSolar), and a radius five times larger than the Sun (R = 5 × RSolar). This star lies along the Main Sequence, where most stars (including the Sun) are found. The fairly high temperature indicates quite blue (hot) colours. You may have observed the star Bellatrix in the constellation Orion through a telescope – this is the region in which it is located.

Try to read the values of L, T, and R for yourself from the diagram. Do you estimate values for the luminosity, temperature, and size of the star similar to those listed above?

Hertzsprung-Russell Diagram. The x-axis is labeled 'Surface Temperature' (in units of kelvins) with high temperatures around 60,000 on the left and low temperatures around 3,000 on the right. The points representing stars which appear furthest to the left are drawn in blue, those in the middle temperature range are drawn in yellow and orange, and those furthest to the right are drawn in red. The y-axis is labeled Luminosity (in units of solar luminosity), with low luminosities around 0.0001 at the bottom and high luminosities around 200,000 at the top. A third parameter, Radius (in units of solar radii), is also labeled. Lines of constant radius extend from the upper-left to the lower-right, covering the whole space with a set of parallel lines. In the lower-left corner we find the line labeled 'R is equal to 0.001 solar radii'; successive lines are labeled 0.01, 0.1, 1, 10, 100, and 1,000 solar radii, with the line for 'R is equal to 1,000 solar radii' being located in the upper-right corner. A series of blue, yellow, and orange points scattered along the 'R is equal to 0.01 solar radii' line is made up of white dwarf stars. A large set of red (and a few yellow and orange) points made up of giant stars appears between the 1 and 1,000 solar radii lines. The 1 solar radii line extends from the upper-left corner to the lower-right corner. The Main Sequence (a curved sequence of blue, yellow, orange, and red points) mainly follows the 0.1 to 10 solar radii lines; at the high luminosity end it curves up to slightly higher luminosities and at the low luminosity end it curves down to slightly lower luminosities. In addition, there are three green lines on the figure which intersect around the point near to 20,000 kelvins and 3,000 solar luminosities. One points down to the x-axis, one points left to the y-axis, and one is parallel to the black lines of constant radius (being drawn at a radius of around 5 solar radii).

How can we find the exact temperature T of a star in this region of the Hertzsprung-Russell diagram, if we know its radius R and its Luminosity L?

We can use the Stefan-Boltzmann Law to relate the temperature (T), size (R), and luminosity (L) of a star to each other. Measuring L, R, and T in solar units, we say that:

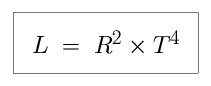
Equation: L is equal to R-squared times T-raised to the fourth power

The next step is to calculate the temperature T, from L and R. We begin by solving the Stefan-Boltzmann equation for T. (We need to have T alone, on the left hand side of the equation.) Dividing both sides of the equation by R2,

Equation: T-raised to the fourth power is equal to L, divided by R-squared

The next step is to take the fourth root of each side of the equation:

Equation: The fourth root of T-raised to the fourth power is equal to T, which is equal to the fourth root of ( L, divided by R-squared ), which is equal to the fourth root of L, divided by the square root of R

Let us say that the luminosity L of the star is exactly 3,270 times that of the Sun. This is just a way of stating how bright the star is relative to the Sun. (For example, if the star is three times as bright as the Sun, L = 3 × LSolar. If the star is one-third as bright as the Sun, L = 0.33 × LSolar.) Similarly, assume that the radius R of the star is exactly 4.56 × RSolar. Now we can plug in the values for L and R into the equation, to determine T.

Equation: T is equal to the fourth root of 3,270, divided by the square root of 4.560, which is equal to 3.53 in units of solar temperature (3.53 times at hot as the Sun).

We have estimated a temperature of 20,000 K for stars found in this area of the Hertzsprung-Russell diagram. To express this temperature in kelvins rather than solar units, we need to compare the temperature of the star to the temperature of the Sun (5800 K). To do this, we just multiply the temperature of the star (in solar units) by the temperature of the Sun.

Equation: T is equal to 3.53 in units of solar temperature, which is equal to 3.53 in units of solar temperature times 5,800 K divived by 1 in units of solar temperature, which is equal to 20,500 K.
(This is an excellent match to our estimate!)