Initial conditions were generated using PMpowerMp code. In this case we use table generated with CAMB code (pk_cambWMAP.dat), courtesy of Andrey Kravtsov.

Initial rms of density fluctuations at z=80.0 in a 250Mpch box is deltaRho/rho =0.0856 for sigma8=0.820 and 2048**3 particles (Nyquist frequency defines upper cutoff of the spectrum with the low cutoff being the fundamental mode). The code uses the Zeldovich approximation, which provides accurate results only if density perturbation is less than unity. This should be valid at any point in space. In practice the fluctuations must be even smaller than unity for high accuracy. With 2048**3 =8G independent realizations of the density, one expects one particle in the box to have 6.5sigma fluctuation, which gives one particle with density 0.55. The first most dense 100 particles are expected to have density contrast 5.76*0.0856 = 0.49.

In practice, we used N=4096**3 particles to make realization of the spectrum of fluctuations with a small correction in the amplitude of perturbations (rms =0.09957) and selected every 8th particle. This serves two purposes. In future we can re-run some fraction of the computational box with 8 time more particles while preserving the same realization of waves. Selecting every 8th particle also slightly boosts the fluctuations close to the Nyquist frequency, which CIC schemes of density assignment tend to dump.

In Start one can find InStart.dat file, which was fed to PMstartMp to set initial conditions. Set of random numbers is provided by file Seeds.1.4096.09781091.dat

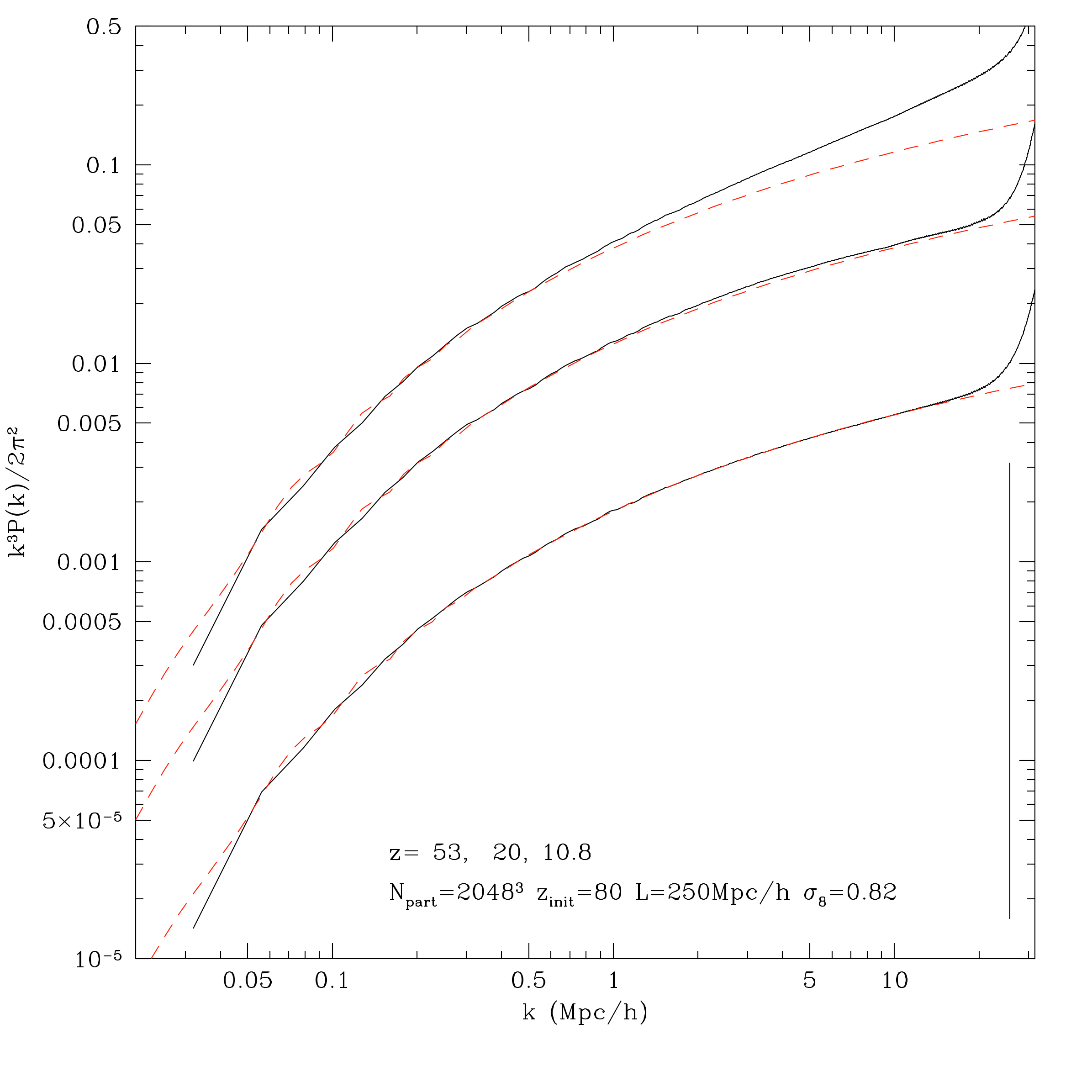	Evolution of power spectrum at early stages. Vertical line at the right is the Nyquist frequency of particles. The spectrum was obtained using CIC density assignment on 4096 mesh. This implies some suppression of power at high wave numbers (about 5-10% at Nyquist frequency)
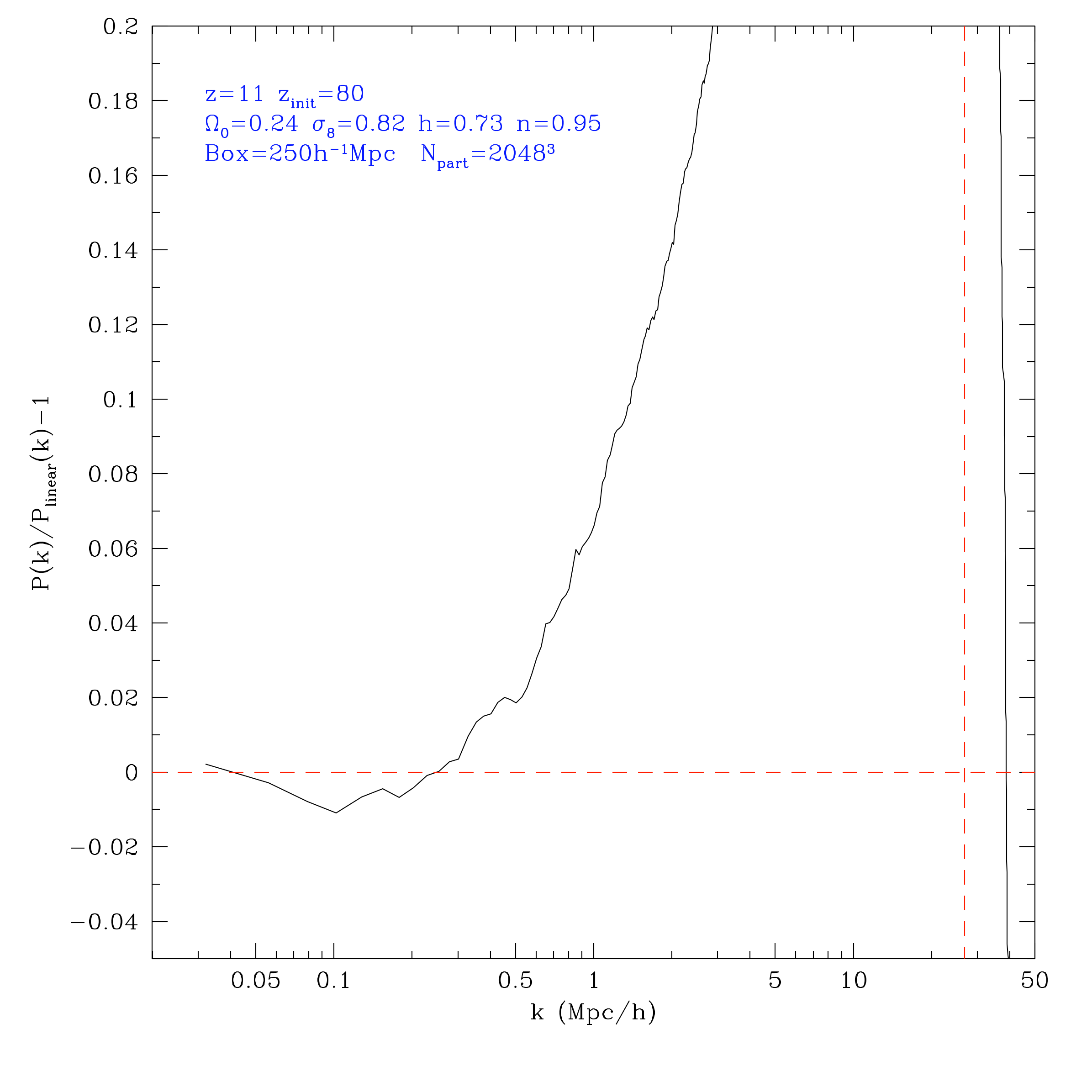	Power spectrum at z=20 (blue dash) and z=11 (black full). Small deficiency of power at k >10 is due to small (4096) mesh: CIC suppresses P(k).
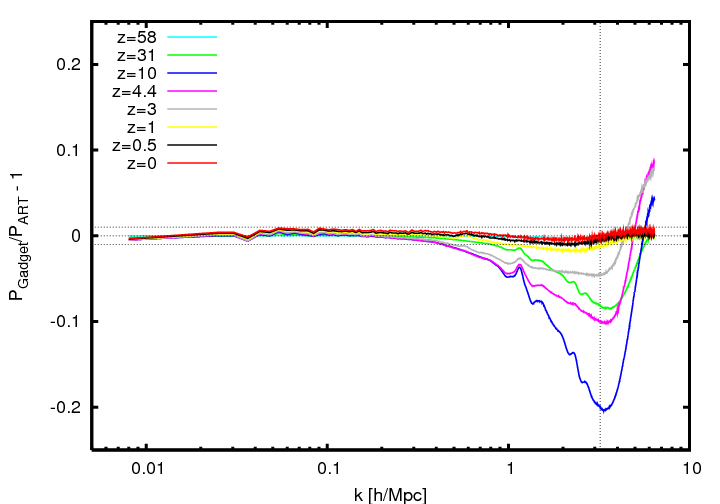	Comparison of P(k) evolution in ART and Gadget-2 codes. This is for 1Gpc/h box with 1G particles. The same initial conditions were used for the runs. The Gadget run and the plot was produced by Ch.Wagner. The vertical line is the Nyquist frequency of particles. Gadget suppresses the growth of fluctuations at high frequencies by 5-20%. The difference gets smaller at low redshifts and practically undetected at z<1.